About focusing
Anyone who has ever taken a photograph knows the importance of focusing. The image projected by the objective gets sharp at a certain distance behind the objective. This distance depends on the focal length of the objective and the distance between the objective and the subject. The device used for recording the image -be it traditional photo-emulsion or digital sensor- should be positioned exactly in the appropriate distance from the objective, if proper image sharpness is required. There are numerous tools and aids in photography that helps finding the proper distance, like frosted glass, focusing screen or up to date auto focus systems. But what's the case with astrophotography?
In astrophotography the main issue is the limited amount of light coming from the subject, often several hours of integration is needed to record the subject appropriately. In such circumstances visual focusing is not possible. In the viewfinder of the camera (if it has any) only the brightest stars are visible, which are point light sources not surfaces with details that could aid the eye to find the accurate focus. Focusing screens also require bright surfaces with details ideally containing edges perpendicular to the edge of the focusing screen's wedge, which is also not given in case of stars. Ordinary auto focus systems fail too because of lack of light and details in the field of view. However point light source characteristic of stars is also an advantage that could be used to achieve perfect image sharpness.
Utilising the phenomenon of diffraction, the light coming from stars can be turned into a focusing aid. Diffraction happens when light closely passes a straight edge, the trajectory of light is bends in the direction of the edge. De amount of bending depends on the wavelength of the light and the distance to the edge it passes. This phenomenon causes the cross shaped spikes around stars in a Newtonian reflector (when light passes the diagonal spider vanes). Putting a mask with appropriately arranged edges in front of the telescope can result a diffraction pattern that can indicate the accuracy of focusing.
Before the actual mask types can be evaluated, it is necessary to determine how precisely shall the distance between the sensor and the objective set, to prevent the error causing noticeable degrading in image quality. The threshold depends on two factors: the focal ratio of the objective, and the resolution of the recording device (grain size in case of emulsion, and pixel pitch in case of digital cameras). One criterion could be the limitation of the diameter of a star's image to be smaller than the pixel pitch. A simplified formula can be used here:
T=F*P
where T stands for tolerance, F is the f/stop of the telescope and P is the pixel pitch. For example in case of an F/4 telescope with a camera having 4.7 microns pixel pitch, the tolerance is only 18.8 microns. This is quite a small value, which increases the importance of a good focusing aid.
The diffraction patterns shown below was calculated using Niels Noordhoek's Maskulator, a wonderful program capable of simulating any kind of masks. Three patterns were calculated for each of the masks, one in precise focus, one with 12 microns off focus, and one at 36 microns. The telescope parameters were set to be a 10" at F/4.
The Bahtinov mask
| ||
 Perfect focus |  12 microns off focus |  36 microns off focus |
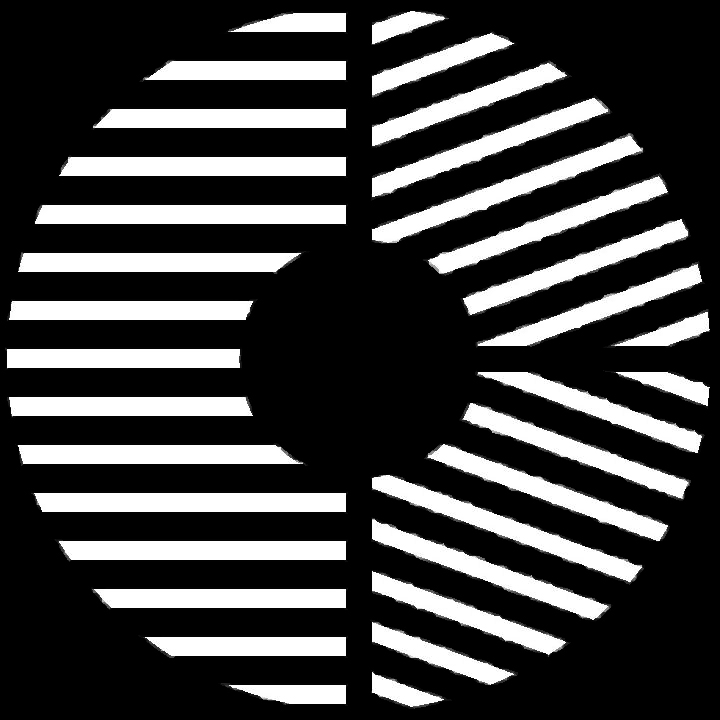The one of the most widely used focusing aids was invented by Pavel Bahtinov. There are slits pointing three different directions in the mask in such a way that one of the directions is parallel with the bisector of the other two. The great advantage of the mask is that it indicates not only the amount of the error, but also the direction of it. Three spikes can be observed in the diffraction pattern, in case of perfect focus they intersect each other in one single point, while as the error increases they move away from that point. Depending the direction of the error the middle spike moves to one side or the other. This feature can be used to determine the direction of the required correction. In practice it is advisable to concentrate on the first bright nodes in the three spikes instead of the spikes themselves, because it is easier to notice small displacements of them.Hartmann mask with two circular holes
| ||
 Perfect focus |  12 microns off focus |  36 microns off focus |
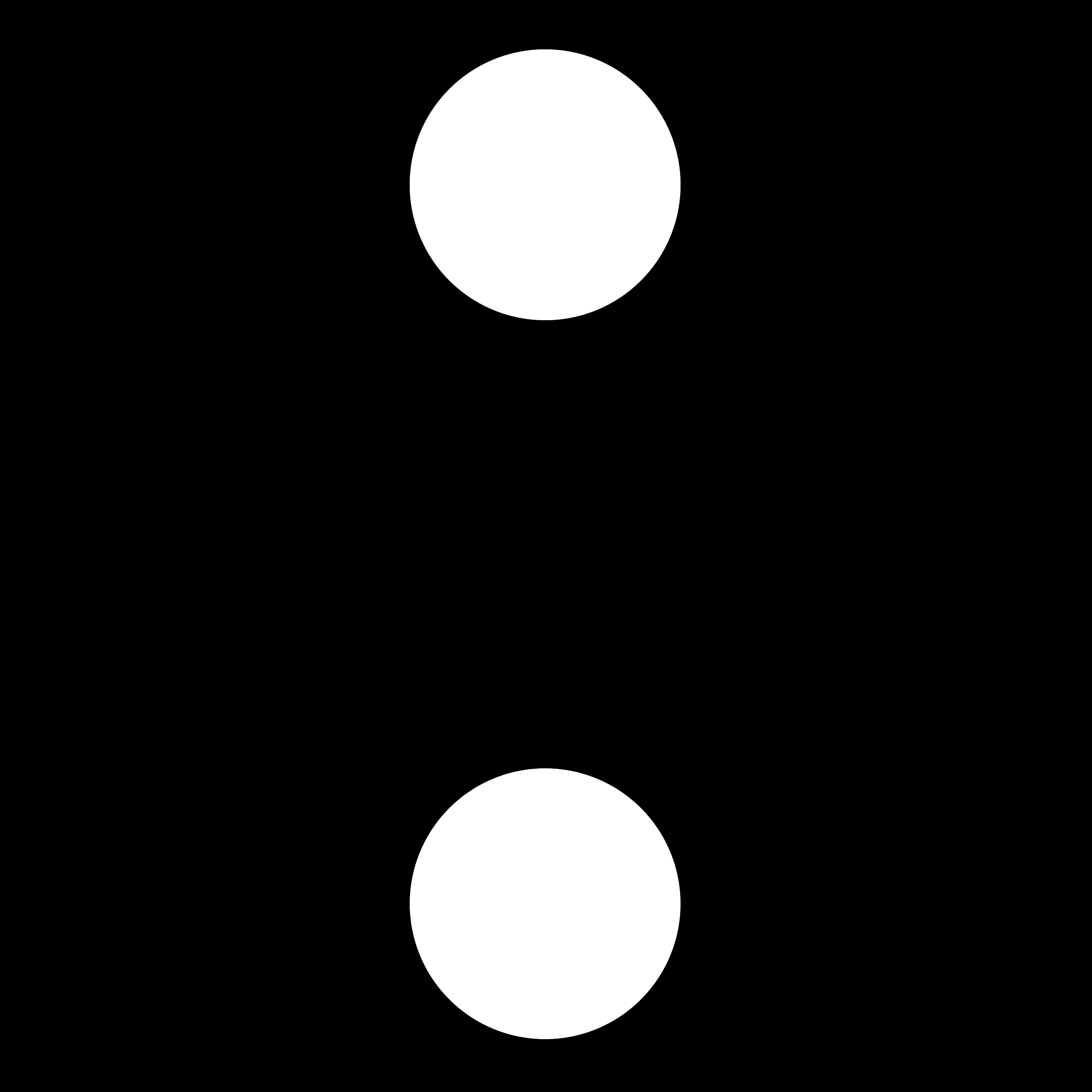This kind of mask is also very common amongst amateur astrophotographers. This one is an exception, because it does not use diffraction, but it creates two light cones instead of one, having the tip of the cones meet exactly in the focal plane, at the location of the star, while their axes are not parallel. In case of perfect focus the image of the star is a point, while off focus the star's image starts to stretch, and at greater errors it separates into two distinct discs. This mask does not indicate the direction of the error. Examining the images below shows that an error of 12 microns can't easily noticed with this mask.Hartmann mask with three equilateral triangles
| ||
 Perfect focus |  12 microns off focus |  36 microns off focus |
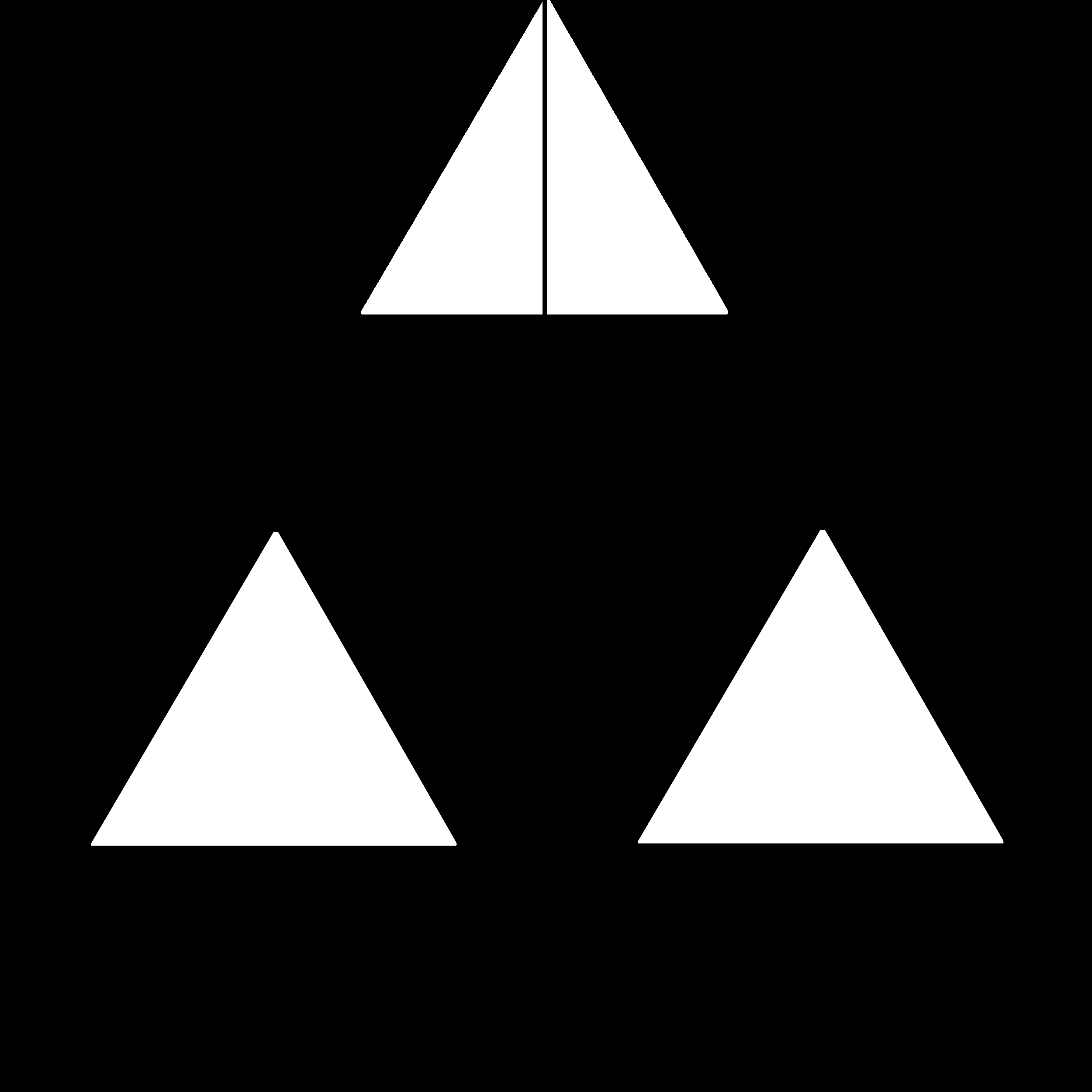This mask, like the previous one, multiplies the number of light cones, and because it also has straight edges it causes diffraction spikes too. The vertical edge in the upper triangle is modelling one the spider vanes in a Newtonian telescope. Because at every angle of the mask one or more spider vanes are visible through the holes, it is recommended to position the mask in the way shown. This way a fourth spike will be created perpendicular to the spike created by the base edges of the triangles. The pictures below shows that this kind of mask is also not so good at indicating small error.The Carey mask
| ||
 Perfect focus |  12 microns off focus |  36 microns off focus |
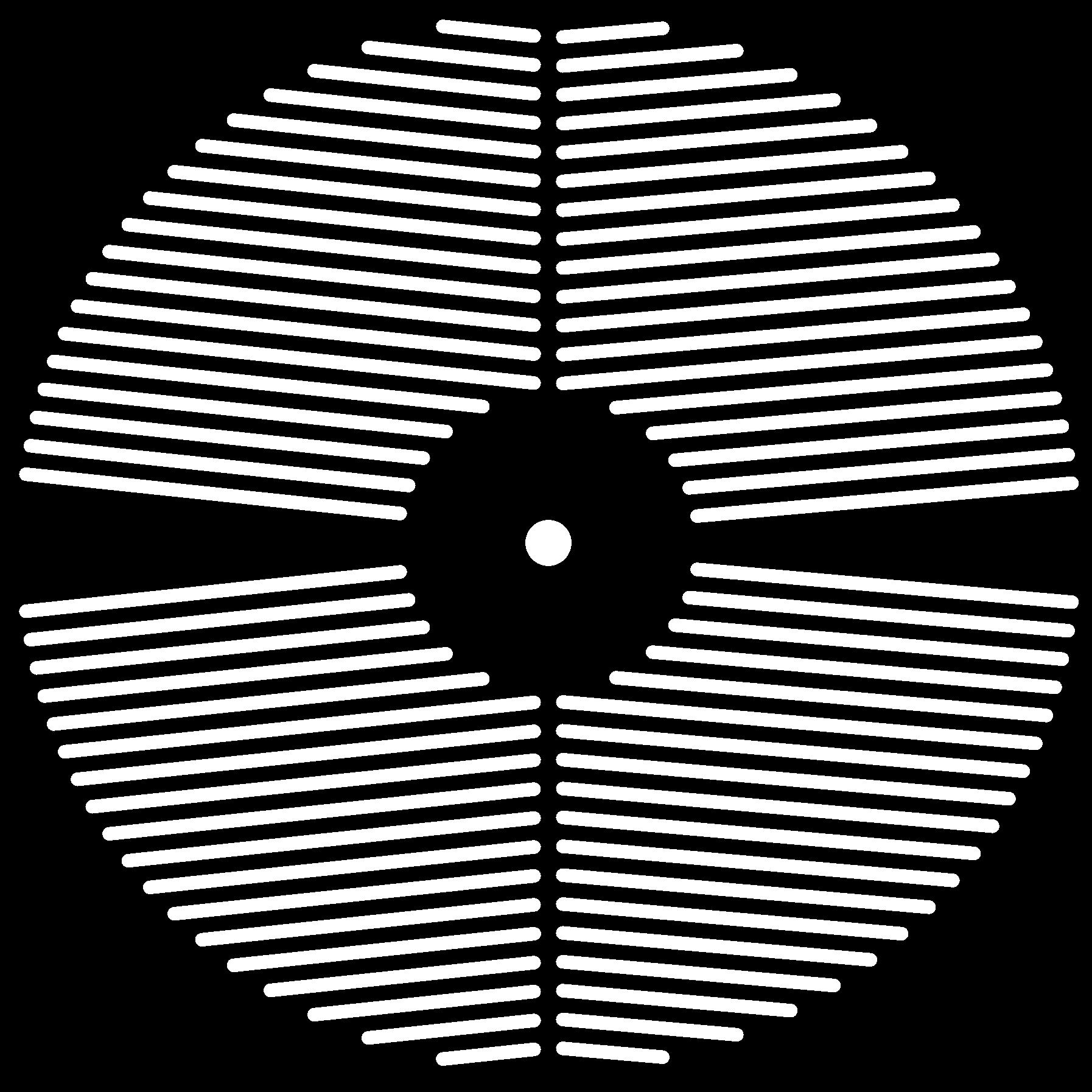This mask utilizes diffraction. The angle between the slits on the left is 12 degrees, while the angle between the slits on the right is only 10 degrees. Both sides creates an X shaped diffraction pattern with the corresponding angle between the lines of the X-es. In perfect focus the intersection points of the X-es overlap with each other, and by increasing the error they are moving away from each other. Because there is only 2 degrees between the spikes of the two X-es, even a small displacement can be spotted, especially a bit farther from the intersection points.The perpendicular mask
| ||
 Perfect focus |  12 microns off focus |  36 microns off focus |
This mask is less known by amateur astronomers. During its construction the main goal was to maximise the displacement of the intersection points of the spikes as the error is increasing. Visually it is difficult to spot small errors, but if the diffraction pattern is analysed by software, the intersection points can be calculated, and the accuracy of the focusing can be increased.
